[ad_1]

Acknowledgments:
My thanks go to Professor Hoffie Lemmer, of College of Johannesburg, for illuminating plenty of features of his revolutionary work; and to the Sydney-based cricket biographer, Peter Lloyd for giving me loads of encouragement.
Preface
This essay has its origins a bit over a 12 months in the past when discussing a broad vary of cricketing issues with pal Ashok Roy, over a dinner at Ish Restaurant within the Interior Melbourne suburb of Fitzroy. The query, what must be completed in regards to the remedy of Not Out innings in figuring out batting averages received an airing, and we swapped some preliminary ideas.
Fairly plenty of articles have appeared over the past three a long time addressing this challenge, most of them placing ahead particular options to the lengthy established “official” resolution. My search has revealed 22 revealed articles of direct relevance, scattered throughout quite a lot of journals – nearly all being authored by those that are effectively certified in statistical strategies (typically known as “statistical science”). The 2 most up-to-date proposals appeared in mid-2020 and mid-2021.
Alighting on the primary few articles, I used to be deterred from doing any form of evaluation by what appeared, at first look, extremely theoretical and largely summary remedies by specialist statisticians. Maybe completed purely for the curiosity of fellow specialists, I surmised. Fairly plenty of the 22 articles referred to are literally of this type, which the lay particular person will probably be unable to become familiar with – no less than with out being immersed in statistical textbooks, for which they received’t have the required time.
While not a specialist in statistical strategies myself, data of them has been gained from a course as a part of my undergraduate diploma, and subsequently from what I’ve wanted to grasp and apply throughout a profession as an financial guide (advising governments on insurance policies for land transport and on regulation of service high quality and costs charged by fuel and electrical energy distribution companies).
When between tasks, I nibbled on the mounting assortment and reckoned that I may knock off this task in a few months. It has taken me six of concentrated effort! Roughly half of this time has been spent in understanding what the assorted authors are proposing and their rationale, a lot of the relaxation being dedicated to evaluating the proposed reforms and forming/making use of my suggestions.
A few articles comprise a evaluation of various approaches advocated, however these are condensed in addition to being written in a largely technical method (one in all 7 pages produced in 2011, the opposite of 5 pages in 2016). So far as I’m conscious, within the public area no less than, this essay represents the primary exposé and critique of the principle proposals put ahead which is directed on the lay one who has not more than an elementary grasp of statistical strategies.
| Observe on Terminology and Abbreviations
Early on, the reader will probably be effectively conscious of the repetition of sure phrases. In order to keep away from the tedium, in sections the place a specific time period is used often, that is abbreviated after initially giving it in full. This is applicable to: Not Out Innings: denoted by NOI and (plural) NOIs. Proportion of Innings which might be Not Out – denoted by Prop NOI. Not Out Rating: denoted by NOS and (plural) NOSs. Projected Not Out Rating: Projected NOS and (plural) NOSs – referring to an estimate of the rating {that a} batsman would have gone on to make if he didn’t should retire when Not Out. Accomplished Innings: CI and (plural) CIs – referring to these innings through which a batsman is dismissed. Accomplished Innings Rating: CIS and (plural) CISs – additionally known as Dismissal Rating. Accomplished Innings Common: CIA – additionally known as Dismissal Common. Official or Conventional Common: refers to these batting averages revealed by the cricket institution (Cricinfo, Wisden and suchlike), primarily based on the traditional methodology of calculation. “True” Common: refers to an adjusted official common that’s supposed to symbolize a batsman’s demonstrated functionality relative to different batsmen. ——————————————————————– Imply worth: refers to a easy common of some information. Median worth: refers back to the center of a knowledge collection. That is the worth that splits the info collection into two halves when it’s ordered numerically (from low to excessive, or vice versa). When there are two center numbers, the worth mid-way between them is taken. |
Overview
This essay critiques the principle different approaches which might be advocated within the literature for figuring out batting averages. Every of those approaches is meant to provide averages that stand as a real illustration of a batsman’s demonstrated capabilities. All have arisen in response to what their authors view to be weaknesses of the traditional method and the ensuing units of “official” averages for various codecs of the sport. All besides one of many seven distinct approaches examined have been revealed within the current millennium.
The three approaches which might be of a sophisticated and esoteric nature are examined and evaluated in Half I. That is completed at some size so that lay readers can readily perceive the rules utilized by every creator in formulating their specific proposal for reform and the underlying logic. The 4 significantly easier approaches are thought of in Half II three of which symbolize (important) variations on a primary theme.
In Half III, essentially the most interesting of the array of proposed options are recognized, and a agency suggestion made on a way for common use. That is then utilized to a sizeable pattern of the careers of Check match gamers. The changes implied to their official averages are examined to be able to point out whether or not or not it will be worthwhile making a changeover, or maybe having two parallel units of averages in operation.
The Epilogue supplies a car for creativity by a non-statistician within the type of an eminent thinker of linguistics, Ludwig Wittgenstein. In a state of affairs that unfolds in 1947 at The Oval cricket floor in London, he suggests what quantities to a radical “rebasing” of batting averages.
PART I – PRELIMINARIES & THE RAREFIED SCHOOL
A. The Context
The phenomenon of remaining Not Out is definitely not trivial. Not Out Innings (NOIs) comprise 6-19% of all innings for almost all of First Class batsmen who’ve performed no less than 20 innings. For instance, of the 229 England batsmen who qualify from the initiation of Check matches as much as mid-December 2021, 60% of them fall inside this vary. For near one-quarter of them, NOIs are lower than 6% of all innings; and for one-sixth of them, NOIs exceed 19% of all innings. The Median worth of this massive assortment of batsmen is 10.2%, and a grand common of 12.9%.
Nonetheless, a stronger affect on a batsman’s Conventional Common is the proportion of whole runs coming from NOIs; and this statistic is usually considerably totally different from the proportion of all innings which might be Not Out. As an example, taking the eight Check batsmen commented on later in Components I and II, the 2 statistics are very totally different in 4 instances – Steve Waugh, Andy Flower, Gary Sobers and Jimmy Anderson; and are considerably totally different in one other two instances – Mohinder Amarnath and David Gower.
Main variations exit within the proportion of runs derived from NOIs for individuals who occupy the highest, center and decrease elements of the batting order. Kartikeya Date has offered an attention-grabbing breakdown of this for Check matches since their inception in 1877 via to early-2014. At this extremely mixture stage, there’s a sturdy development in each the proportion of NOIs and the incidence of Not Out runs as one strikes down the order, with a marked rise for the final three positions – particularly for the quantity eleven place.
| Place | % of NOIs | Not Out Runs per 1,000 Runs Scored |
| Opener | 5.1 | 1.5 |
| Three | 6.3 | 1.7 |
| 4 | 7.8 | 2.0 |
| 5 | 9.2 | 2.7 |
| Six | 9.6 | 3.3 |
| Seven | 10.9 | 4.5 |
| Eight | 13.3 | 7.2 |
| 9 | 15.6 | 11.8 |
| Ten | 25.1 | 29.1 |
| Eleven | 47.6 | 105.3 |
As to be anticipated, it’s common to search out massive variations between particular person batsmen occupying every of those positions.
Historically, NOIs have been considered a free finish, to be handled by an simply utilized tidying-up conference. There isn’t a express rationale for this conference, solely an implication that it’s illogical to treat all NOIs as being topic to imminent dismissal.
However why such curiosity in – and all of the fuss over – how Not Out Innings are handled in compiling batting averages? One wants to remember that the normal variety of runs made per accomplished innings is essentially the most generally used single determine indicator of batsmen’s comparative high quality of efficiency and thereby additionally of their demonstrated relative functionality. Varied causes have been put ahead for wanting an improved remedy:
- The traditional method, in impact, provides on to every Not Out Rating (NOS) the typical of a batsman’s Dismissal Innings Scores.[i] An implication is that the batsman would, if allowed to proceed his innings, have gone on to achieve that enhanced rating. That is price noting in itself, and in addition as a result of a lot of the proposed options to coping with NOSs are involved with projecting them, in a method or different, in order to be equal to – and have the identical standing as – really accomplished innings.
- Some gamers artificially enhance their common with NOSs which might be within the neighborhood of, and typically exceeding, the perfect scores they’ve been in a position to obtain of their Dismissal Innings.
- In instances the place a batsman’s NOIs are frequent and his NOSs are comparatively excessive, it will are likely to distort his common as an indicator of efficiency and functionality.
- The Conventional Common can, in precept, exceed a batsman’s highest Dismissal Rating even when that rating is higher than his highest NOS.[ii]
As has additionally been identified:
- Many batsmen at numbers 8-11 within the order are denied the opportunity of materially advancing their common by their lowly place, being allowed to scrape collectively only a few runs whereas their companions – missing religion in them – not solely farm the bowling however play cavalierly and go away their associate stranded on a low NOS.
- Conversely, some low-in-the-order batsmen make their status for having a good common by being stranded on this approach, but hardly ever if ever making a considerable rating.
- Excessive NOSs are sometimes gained by center order batsmen who’ve had ample time on the crease to compile a great rating earlier than a workforce declaration is made.
In assessing the assorted proposals for reform, I’ve had two foremost issues in thoughts:
Relevance to the underlying downside and the character of the rationale offered for the proposed reform.
Intelligibility: are lay individuals, as potential customers of the outcomes, in a position to readily comprehend a given proposal and the way it’s derived? If they’re unable to become familiar with it, they could regard it with some scepticism or suspicion, and so be reluctant to belief the outcomes obtained from making use of it.
In consequence, some would possibly sympathise with the catch cry of Benjamin Disraeli (British Prime Minister from 1874-80): There are three sorts of lies: lies, damned lies, and statistics. He would even have meant statistical fashions.
Because the second of those exams represents a possible hurdle, particularly within the case of approaches mentioned in Half I, a essential process is the try made to demystify them.
Faculties of Thought
The proposed strategies fall into two distinct camps, which serves to organise the dialogue:
The Rarefied Faculty
- These proposals bear the hallmark of nice consideration to theoretical correctness and have a sophisticated derivation, therefore are of an esoteric nature.
- Essentially the most primary ideas are usually laid out as equations. As an example, the normal common (whole runs scored divided by variety of accomplished innings) is specified, in alternative routes, as:
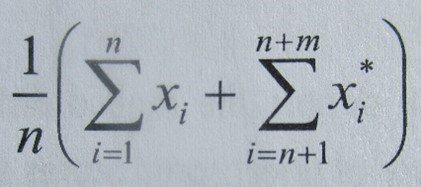
During which: x 1, 2,…n denote the runs scored by a batsman in n accomplished innings, and x*n + 1, n + 2,…n + m denote the runs scored in m not out innings.

During which: x1, x2, …, xm denote a batsman’s dismissal scores, and x*m+1, x*m+2, … x*n denote his not out scores.
Relaxation assured, there’s not one other mathematical equation in the remainder of this essay – solely three comprehensible components, written in phrases.
The Simple Faculty
- These proposals tackle the issues at hand with a lot much less elaborate or difficult strategies (as examined in Half II).
B. The Rarefied Faculty
Pioneers
There are three distinct and contrasting approaches to think about. The primary is a seminal contribution made by Alan C. Kimber and Alan R. Hansford in an article of ten and a half pages (plus two appendices), showing in 1993 within the UK’s prestigious Journal of The Royal Statistical Society (JRSS). It tremendously stimulated curiosity within the matter of how Not Out Scores must be handled in arriving at averages, and in addition within the related challenge of the everyday distribution of batting scores (from low to excessive, or vice versa). While the Kimber-Hansford article is, in the principle, extremely technical, how and why they arrive at what’s advocated will be defined in non-technical phrases.

Alan Kimber – seminal considering
All besides one of many 22 articles examined have been written following the looks of the Kimber-Hansford paper. The only real exception, a research by Peter J. Danaher of the College of Waikato (New Zealand), advocated primarily the identical method. Danaher’s temporary article (5 and a half pages) was revealed 4 years previous to that of Kimber-Hansford in a a lot much less recognized journal with a small circulation, the New Zealand Statistician. His article isn’t cited, and it’s clear that Kimber-Hansford had been unaware of its existence once they did their work. I shall return afterward to his research and its classes.
The background of the 2 authors at hand could be very totally different:
- Alan C. Kimber: then a researcher and lecturer in statistical strategies on the College of Surrey, in his mid-30s, with a specialist curiosity in evaluation of information on supplies and tools reliability and within the modelling of survival information regarding illnesses and coverings.
- Alan R. Hansford: a Sussex county participant from 1989-92, when in his early-twenties. He offered the cricket folklore, together with totally different phases of gamers’ careers and the everyday course of an innings, in addition to a lot of the batting statistics.
Accordingly, I shall consult with the proposed methodology of deriving batting averages as that of Kimber. In impact, he {followed} on from the place George Henry Wooden left off half a century earlier. Wooden was an eminent labour market statistician, commerce union activist and a extremely educated cricket fanatic. His paper titled Cricket Scores and Geometric Development was learn earlier than members of the Royal Statistical Society at its premises within the coronary heart of London in November 1944, Wooden then being in his early-seventies. It was revealed, together with an account of the next full of life dialogue, within the Society’s JRSS journal the next 12 months (shortly earlier than his loss of life).
Wooden was sceptical of acquired opinion that batsmen’s scores made in accomplished innings show a “geometric development”. By that is meant they show an roughly mounted sample, through which the variety of scores achieved by a batsman sometimes scale back by an roughly fixed ratio because the scores improve in magnitude from zero. The scale of this fixed tends to vary, usually considerably, between one batsman and one other.
Two examples of roughly geometric progressions are offered under, with the variety of accomplished innings being given as a frequency distribution.
| G. HAYWARD | F. SIEBEL | |
| Variety of | Variety of | |
| Runs Scored | Accomplished Innings | Accomplished Innings |
| 0-19 | 53 | 64 |
| 20-39 | 36 (0.68) | 34 (0.53) |
| 40-59 | 24 (0.67) | 19 (0.56) |
| 60-79 | 18 (0.75) | 9 (0.47) |
| 80-99 | 11 (0.61) | 4 (0.44) |
| 100-119 | 8 (0.73) | 2 (0.50) |
| 120-139 | 6 (0.75) | |
| 140-159 | 4 (0.67) | |
| Ratio of decline in variety of innings: | Approx 0.7 | Approx 0.5 |
A geometrical sample happens if a batsman is equally more likely to improve his rating by a small increment (notionally, by one run) no matter rating he then occurs to be on. In different phrases, his probability of being dismissed at any time throughout an innings could be unbiased of the actual rating that he has then reached.

George Henry Wooden who began all of it
George Wooden examined the scores of three “massive teams of batsmen over very many innings” (all English gamers at First Class stage) and located materially decrease survival charges within the 0-5 run vary than past, in addition to the presence of fairly extra innings of 80-100 and upwards, than is implied by a geometrical development. He concluded that such a development applies “in the principle to batting scores”, conforming to it over the “main half of their vary”. Nonetheless, he additionally thought it possible that the noticed higher issue of scoring very early on in an innings, and the far higher ease of scoring when effectively established, are common and inherent options of the sport. Varied potential causes occurred to him for the existence of those options and he was eager for these to be rigorously examined.
Alan Kimber took up the reins and thoroughly examined for the existence of a geometrical development of batting scores at First Class stage, with its key characteristic of roughly fixed danger being confronted by a batsman as his innings rating progresses. The relevance of the talk as as to whether or not such a development really holds true, and its consequence that accomplished scores would are usually roughly evenly distributed from low to excessive, is as follows. If true, this may indicate {that a} specific estimation process is legitimate for arriving at an estimate of the variety of runs a Not Out participant would have finally scored had he batted on. The issue at hand would, in impact, be solved!
| For these interested by Alan Kimber’s approach of probing this challenge and in figuring out a number of the element of his findings, that is offered instantly under. Different readers can skip to the part following, on his method to estimating a batsman’s “true” common. |
Probing Cricketing Folklore
Kimber carried out his empirical exams for the existence of the geometric phenomenon after first noting that the acquired cricket knowledge – that’s, widespread cricketing beliefs going again an extended whereas which might be presumed to be true – is in distinction with it. The acquired knowledge is that batsmen are usually most susceptible when beginning their innings, and if survive lengthy sufficient – ie via a playing-in interval – they turn out to be effectively set (although typically affected by nervousness when approaching milestones). However after reaching a extremely huge rating (100 plus), sometimes doing so after some hours of batting, they have an inclination to tire and turn out to be extra error susceptible, and so turn out to be extra liable to be dismissed.
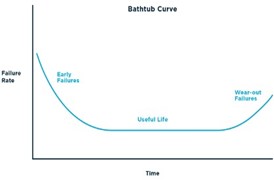
In view of this cricketing folklore, Kimber suspected that the distribution of batsmen’s scores will sometimes approximate to a bathtub form (as depicted under) – drawing on a fairly tenuous analogy from his familiarity with reliability engineering research, particularly charges of malfunction and bodily failure over time of kit parts. Making use of this analogy to batting, the “failure price” turns into the speed of being dismissed when on totally different scores, and “the passage of time” turns into scoring of accelerating magnitude. Within the drawing under, the playing-in, well-established and fatigue phases of an innings would exchange the phases famous in blue script.
In inspecting which of the 2 variations is the case, Kimber focussed, firstly,on the proportions of profession scores manufactured from zero by 9 batsmen in Check matches, by 2 batsmen in different First Class matches and by 2 batsmen in One-Day Internationals (ODIs) – al advised spanning 5 nations. The noticed proportions are discovered to be at all times increased than these implied by the geometric mannequin: for 9 of those 13 batsmen it’s 2.4 to 4.6 instances increased.
Secondly, he examined the sample of excessive scoring dismissals made in First Class matches by 4 prolific centurions (Victor Trumper, Don Bradman, Mohinder Amarnath and Dennis Amiss). The developments are clearly obvious after smoothing the innings-by-innings information plots (via making use of a type of shifting common).[iii] Though the info are more and more sparse within the higher tail of the scoring distributions, dismissal charges do appear to rise considerably in three of those 4 instances – doing so after 70 runs for Amarnath, after 120 runs for Trumper, and after 230 runs within the case of Bradman. However the rises in dismissal charges are small, and the profile is taken into account to be “fairly flat at their excessive scores, and it appears believable that the tail of the underlying scores distribution for a batsman is no less than roughly geometric” – ie a roughly fixed diploma of danger is encountered.
Thirdly, Kimber utilized a normal statistical check of how effectively the geometric development mannequin matches the noticed distribution of scores (a “goodness of match” check). For this, he takes a random pattern of twelve units of profession scores (some for Checks, others for ODIs),[iv] and in addition 4 units of century scores in First Class matches for Jack Hobbs, Colin Cowdrey, Glen Turner and Dennis Amiss (all with greater than 100 centuries to their title). This confirmed sturdy proof in opposition to the geometric development speculation on the low scoring finish. There was weaker proof in opposition to the speculation within the higher tail – with some, although not pronounced, assist for batsmen being extra liable to dismissal on the very excessive scoring finish.
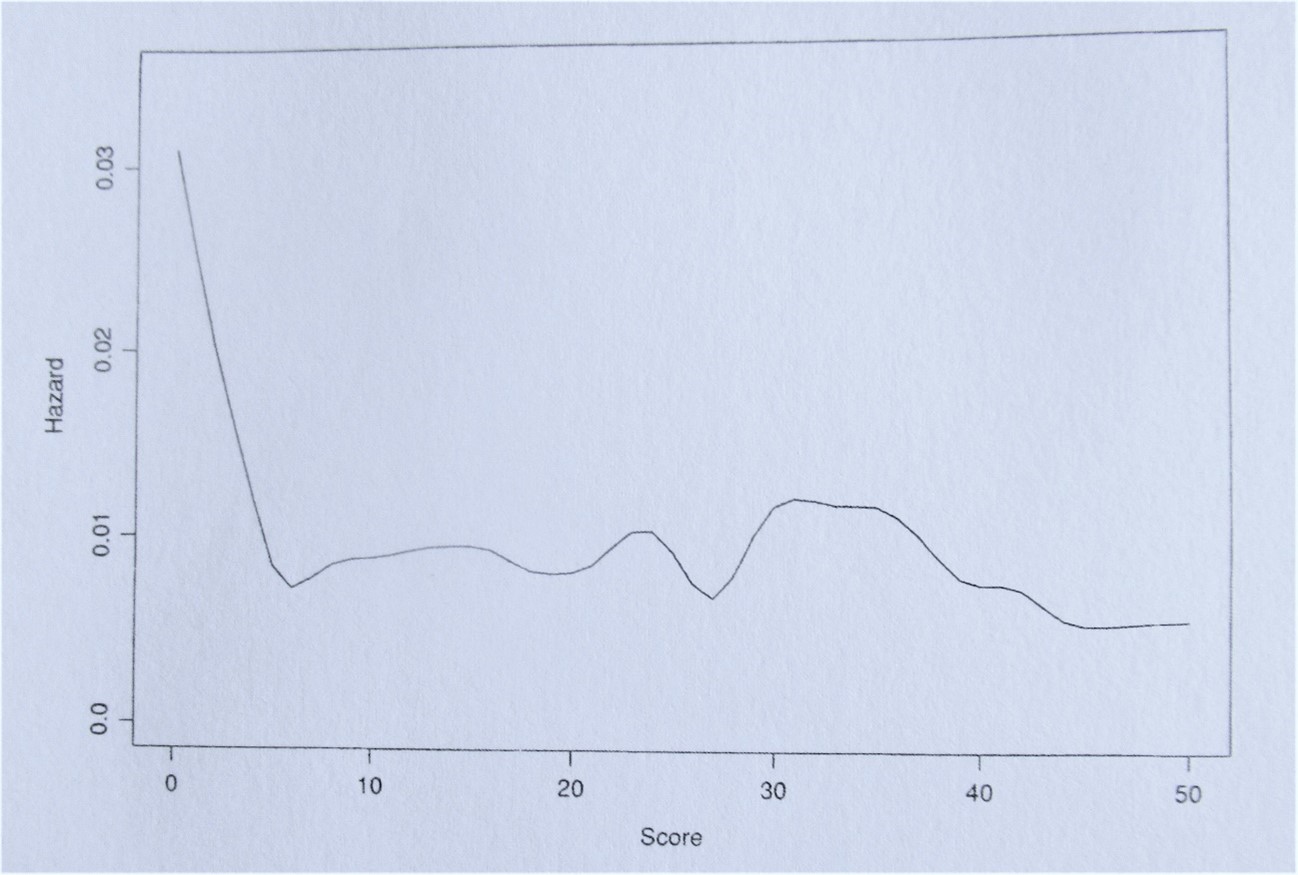
As with the surmised bathtub curve, comparatively excessive dismissal charges happen in the course of the preliminary few runs of an innings, then falling sharply to enter an extended flattish section with none persistent underlying pattern; with dismissal charges seeming to rise from across the 130-150 run mark. On the low finish, the graphs introduced point out that the magnitude of danger (or “hazard”) encountered begins to flatten out for Dennis Amiss at round 10 runs, and for Victor Trumper and Don Bradman at round 7 runs.
The form of Bradman’s danger plot regarding scores of as much as 50 in his First Class innings is proven under (after the info is smoothed):
Kimber has now amply confirmed George Wooden’s scepticism about notions of a geometrical development. Accordingly, if an enchancment is sought on the historically decided batting common, a distinct methodology should be employed. The preliminary work simply described constituted the bedrock for Kimber shifting ahead, and it takes up 4 and a half pages of the article.
Alan Kimber’s Strategy of Figuring out a Batsman’s “True” Common
Kimber arrives at an estimate of a batsman’s actually consultant common in two foremost levels:
– Making use of a mechanism often called the Product Restrict Estimator, which is an inherently probabilistic method to projecting incomplete information to notionally concluded outcomes, the unfinished information right here represented by a batsman’s Not Out Scores. This mechanism is his lynchpin.
– Utilizing the ensuing set of projected Not Out Scores, plus the recognized set of Dismissal Scores, to ascertain a batsman’s true common.
(i) The Product Restrict Estimator Defined
This extensively used instrument had been devised in the course of the Nineteen Fifties by two American researchers, Edward L. Kaplan (a mathematician) and Paul Meier (a bio-statistician), their work being revealed in 1958 in a now well-known article.[v] Considerably, the Product Restrict Estimator (PLE) had its origins within the context of medical follow-up research of a pattern of sufferers, to point out how efficient a specific remedy (or kind of surgical procedure) is in contrast with software of a distinct kind of remedy or taking no motion. Two-thirds of Kaplan-Meier’s checklist of 28 references relate to papers on life expectancy, survival charges for illnesses and after remedies, and follow-up well being research.
In brief, these two researchers created a statistical methodology to find out survival charges (depicted as survival curves) for teams of sufferers when the accessible information was topic to incomplete observations of their post-treatment progress. To cite The New York Occasions in 2011:
“The Kaplan-Meier curve has turn out to be the usual instrument utilized by medical researchers for figuring out the period of survival in 1000’s of research, starting from most cancers to AIDS to heart problems to diabetes, to call only a few.”
In monitoring the experiences of a pattern of handled sufferers, there usually arises the necessity to fill gaps created by those that have dropped out of a follow-up research aimed toward establishing the possible timing of relapse (or loss of life) following a remedy or surgical procedure – reminiscent of some type of most cancers remedy or kidney substitute. Such sufferers are misplaced to the research both attributable to their deliberate resolution to now not take part or from inadvertent lack of contact. The long run course of their situation is in precept discoverable however is unknown – hidden from view, fairly than being revealed – and they also turn out to be lacking from the general information set. The information collected previous to a affected person dropping-out is, fairly oddly, termed censored information.[vi]
Initially, the PLE’s preliminary position was to ascertain an anticipated (most probably) eventual end result for drop-out sufferers and thereby allow the creation of a sound, complete, information set for the affected person pattern as an entire. These working a research would then in a position to make dependable statements in regards to the possible survival prospects of future sufferers.
Basis Ideas
The PLE mechanism is based on three foremost rules:
(a) Being misplaced to a follow-up research is assumed to be a random occasion, unconnected with the expertise of different sufferers being monitored. For cricket, the counterpart assumption is that the incidence of retiring Not Out will not be influenced by any of that batsman’s beforehand accomplished innings.
(b) A affected person having an uncompleted remark in the course of the follow-up course of must be considered having the identical prospects of continued survival as these sufferers who proceed to be followed-up – ie all those that haven’t but relapsed (or died).
For cricket, this suggests {that a} batsman who has to retire Not Out would have the identical prospects for continued survival as when persevering with to bat on having already reached that specific rating and in the end completes these innings (be dismissed). In brief, a batsman’s Not Out innings is handled as being represented by the expertise of the array of his surviving innings at and past that rating – which Kimber notes “is an affordable assumption”. So he has regard needed to all dismissal scores of a batsman that equal and exceed his Not Out rating in query.
(c) In monitoring sufferers’ post-treatment progress, the survival prospects of members who’re recruited early and afterward into the research are handled as being instantly comparable. In different phrases, it’s reliable to deal with later joiners in the identical approach as early joiners, and that the timing of becoming a member of itself is assumed to not have any affect on a affected person’s survival prospects.
For cricket, this suggests projecting a batsman’s Not Out rating close to all innings performed in the course of the course of his profession, or season, or the actual collection of matches being examined. That’s, taking account of the scores {that a} batsman makes each previous to, and after, a given Not Out Innings has occurred.
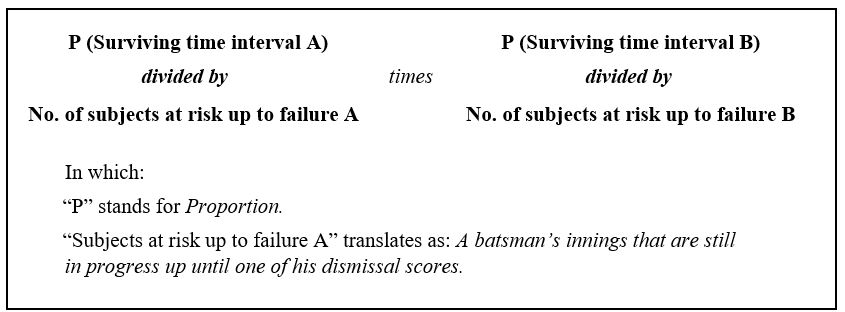
A totally labored instance of the PLE’s use in survival evaluation is offered in a readable article by Ilker Etikan and colleagues (revealed in 2017). They reproduce the important thing components for arriving on the anticipated time to relapse (or loss of life) of a drop-out affected person, as given within the field under. While the that means of particular person parts of this components is obvious (“topics” are sufferers, and “failure” is a relapse or loss of life), the mechanics of working the components is a extremely difficult affair. Tailor made pc software program is obtainable commercially to function it, and so the components will be utilized by a lay particular person whereas being blissfully unaware of the mechanism churning away!
In spirit, the PLE’s method to the gap-filling mission is to base the survival prospects of a drop-out affected person on the noticed survival charges (instances to relapse or loss of life) for every of all the remainder of the sufferers who’re persevering with to be monitored. The essential process is to establish the proportion of those sufferers who cross a given variety of time intervals (reminiscent of entire months) earlier than then relapsing (or dying), and so exit the monitoring train at that time.
The chance of a drop-out affected person relapsing instantly upon crossing a given time interval will be estimated instantly from the observations made on the speed at which of different sufferers relapse with the development of time following their respective remedies. Therefore, the anticipated time to relapse for a drop-out affected person displays the collection of different sufferers’ relapse instances and their related chances. On this approach, account is taken of their collective experiences.
| For individuals who could also be curious, making use of the PLE’s estimation process is greatest defined close to its use as a part of a “survival evaluation”. Different readers can skip forward to “Kimber’s Adaptation”. |
Additional Element
The next steps are concerned in making use of the PLE to survival evaluation:
- Distinguishing sufferers persevering with to be monitored (ie not but relapsed or died) from those that have dropped out.
- Projecting the size of time that every drop-out affected person will be anticipated to proceed till they are going to relapse or die (ie an estimate of the most probably interval), primarily based on noticed survival charges (instances to relapse/loss of life) for every of the remainder of the sufferers. A month would possibly, as an illustration, be used because the related interval of time.
- The survival charges of the drop-outs is established by:
(a) figuring out the proportion of sufferers who survive (ie go via or cross) successive intervals of time following their remedy with out relapsing (or dying), as illustrated by the diagram under, and thereby
(b) establishing the proportion of sufferers who go via a sure variety of time intervals earlier than then relapsing (or dying) – and so not persevering with past that time.

The above chart is a smoothed model of what, in apply, is a collection of steps – as proven under:
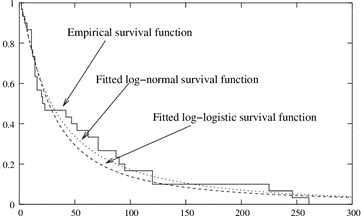
This second set of observations on the speed at which sufferers relapse with the development of time following remedy, is considered as a frequency distribution of instances to relapse. The chance of a drop-out affected person relapsing instantly upon crossing every time interval will be estimated instantly from the relative frequencies. The upper the frequency after a given time frame, the upper the chance of the drop-out relapsing then.
(c) The possible variety of time intervals to the anticipated relapse of a drop-out affected person is given in keeping with the mathematical notion of “anticipated worth”, by multiplying every numbered relapse interval by its respective chance, after which summing the solutions.
The plot of information with a survival curve is akin to utilizing a life (or actuarial) desk which reveals, for a specific particular person reaching a sure age, what the chance is that they are going to die earlier than their subsequent birthday, and therefore their chance of surviving any specified age. Kimber acknowledges that his personal method “could be very a lot in the identical spirit as instructed by Mr G.R. White of a batting common primarily based on a life desk” (talked about in dialogue of G.H. Wooden’s paper given in November 1944).
Mr White put ahead the concept of acquiring a batsman’s true common “by including to the runs really made an expectation of life in every of the uncompleted innings”. Though how a life desk could possibly be constructed was solely tentatively broached, and Mr White himself foresaw the objection that, “One would by no means get the concept over to the general public who comply with the sporting columns of the every day papers”.
In utilizing the PLE mechanism, the presence of knowledge generated a couple of given affected person surviving a sure time frame (analogously, a batsman no less than reaching a sure rating throughout an innings), and about that affected person passing a specific interval of time (analogously, the batsman passing a specific rating throughout an innings) is superfluous for arriving at a batting common. Within the context of the evaluation of sufferers, that is certainly important info. The reason is that GPs and medical specialists even have a a lot stronger curiosity in such info than they’ve in a greatest estimate of precisely when a specific affected person will be anticipated to relapse or die. And the sufferers themselves hardly ever ask their GP/well being adviser to offer them an estimate of precisely what number of extra weeks/months/years they are often anticipated to “survive” – which is simply one of many outputs from the pattern affected person information after the PLE has been utilized.
What sufferers will sometimes need to know is, what their likelihood is of “surviving” (that’s, not relapsing/not dying) for an additional, say, 3 or 5 years – or till they’re, say, into their sixties or seventies. A spot estimate of their possible time to relapse (demise) will anyway, virtually definitely become improper! Though the estimate will be the most probably of all estimates that may be made, it’s very unlikely to exceed a 50% probability of being proper. However for attempting to foretell (foresee) the long run prospects for a given Not Out Innings, a spot estimate of some kind is important!
Kimber’s Adaptation
In imaginative style, Kimber attracts on the PLE mechanism to analyse cricket scores by making the next collection of substitutions:
- the assorted innings performed by a batsman is substituted for the set of sufferers underneath research,
- the development of runs made by a batsman (ie entire numbers from zero upwards) is substituted for the development of time from a specific start line,
- having to retire Not Out (attributable to harm, in addition to occasions exterior to the batsman himself) is put rather than a affected person dropping out of being monitored,
- the incidence of a dismissal is rather than a affected person’s relapse or loss of life,
- a single run scored is rather than the PLE’s interval of time (per week, month, 12 months or no matter); a batsman strikes his rating alongside in runs made, passing from a sure variety of runs made to the subsequent rating. (If he hits a two or a 4, these respectively stand for passing two and 4 intervals.)
Accordingly, in a cricket context, the expectation for a batsman as to the eventual end result of a Not Out Innings (NOI) is predicated on the relative variety of instances he reaches totally different accomplished scores in the course of the totality of his innings being thought of. The related scores for projecting a NOI (these equalling/exceeding it) are depicted as a frequency distribution. The most probably potential end result for the NOI is then primarily based on the mathematical notion of anticipated worth.
This worth is discovered by assigning a chance to every of the assorted accomplished scores of relevance, multiplying their respective chances by their magnitudes, after which summing the ensuing numbers (the “merchandise”).
For instance: a rating of 5 runs that’s made on three events must be given a chance 3 times as nice as a rating of twenty runs that’s made solely as soon as. If a batsman has performed a complete of sixty innings, the chance of being dismissed when on 5 runs is then 0.05 (3/60).
Multiplying this reply by the rating involved (5 runs) offers its contribution to the anticipated worth for the general set of scores – on this instance, 0.05 instances 5, offers 0.25 runs.
This process is repeated for every of the opposite scores in flip, and the ensuing contributions are added to reach on the anticipated worth.
The above process is equal to the extra on a regular basis notion of calculating a weighted common for a set of scores – ie every accomplished rating multiplied by the variety of instances it has occurred, then divided by the full variety of innings performed.
Formally, that is additionally equal to easily including all of a batsman’s particular person accomplished scores and dividing the ensuing sum by the full variety of innings performed – ie taking the Imply worth (arithmetic common) of all his related scores.
After some trial and error, I found that what’s a sophisticated and extremely elaborate process utilized, as by the PLE, can safely be decreased to the next assertion:
- If a given Not Out Rating is projected to its conclusion by taking the Imply worth (arithmetical common) of all really accomplished, and notionally accomplished scores that exceed and equal it, it will produce an an identical outcome to making use of the PLE mechanism.
- The related scores on this context embrace any different Not Out Scores which, when projected, equal/exceed the Not Out Rating being thought of. This iterative characteristic of the PLE (and therefore Kimber’s) estimation process is logical and internally constant. It does introduce an extra complication, however this may be coped with by projecting the best Not Out Rating initially and coping with others in descending order. (It will keep away from an interminable collection of re-workings.)
Proof of this actual correspondence is given by my reproducing Kimber’s estimated “true” averages for six of his batsmen utilizing the simplified process described above.
This discount is fairly like a mansion such because the Palace of Versailles (a former royal residence) being recreated in all its glory although with minimal structural assist. Maybe a number of the skilled statisticians realise this, however are preserving it underneath their hats!
I imagine that when writing the article, Alan Kimber was unaware that the easy methodology simply described equated to his personal when it comes to outcomes, though he would little doubt have tumbled to it if in search of to experiment. For him, it was an attention-grabbing alternative to use methods he was skilled in, and ideas he was extremely acquainted with in his skilled life, to the totally different matter of batting in cricket. And it has turned out to be a one-off foray.
Nonetheless, as mentioned in Half II, use of anticipated values in projecting a Not Out Rating to a notional conclusion will not be essentially the perfect information for prediction, particularly with a reasonable or extremely skewed set of information for a batsman’s scores.
A Particular Case: If a batsman’s prime rating occurs to be Not Out, the estimate to be made by the PLE method is undefined; and so an assumption needs to be made in regards to the lacking higher tail-end of scores. Alan Kimber bases this on proof that, in First Class cricket no less than, this finish of a scoring distribution tends to show a roughly geometric development, and so batsmen have an roughly fixed danger of being dismissed as their rating progresses on this area. Additional, the diploma of danger confronted will not be a lot totally different to that confronted when a batsman has received an preliminary dozen or so runs underneath his belt.
Accordingly, as a common rule, a prime scoring Not Out innings can fairly be projected to an eventual end result by including on the typical of the batsman’s scores above, say, ten runs. (This contains different Not Out Scores at their projected ranges.) For these batsmen who’ve performed no less than twenty innings, the impact on their estimated “true” common will hardly ever be delicate to specifying this mark a number of runs both aspect of the nominated ten.[vii]
It’s famous that plenty of different researchers have subsequently devised survival curves for particular person batsmen in numerous types of the sport. Examples are:
- Bernard Kachoyan and Marc West (2018 article) who generate survival curves for quite a lot of batsmen, indicating their chance of constructing totally different scores at every level in an innings – ie given the variety of runs that he has already scored. In addition they present that these curves will be constructed from abstract info that’s accessible from plenty of revealed cricket information bases. Outcomes are given for the careers of six Check match batsmen.
- Hemanta Saikia and Dibyojyoti Bhattacharjee (2018 article) who create survival curves for batsmen within the Indian Premier League’s 2012 season. Their findings are supposed to be used in arranging the batting order of a workforce enjoying Twenty20 cricket, and in addition for making changes to the batting order throughout play in order to mirror the unfolding match scenario.
(ii) Utilizing the Ensuing Set of Projected Not Out Scores
Having utilized the PLE mechanism, Kimber has a single set of information to work with: the scores of all really accomplished and notionally accomplished innings (one for every innings performed). These numerous scores at the moment are on an equal footing.
On this temporary second stage, these two units of scores are added to offer the full runs credited to a batsman, which is then divided by his whole variety of innings performed – so arriving at his “true” common.
Scope of Utility
Kimber’s methodology is meant to be relevant to entire careers in Checks and in First Class matches usually, and in addition for innings performed in a single season -16 innings being the smallest quantity he experiences findings for, the subsequent being 24 innings. Desk 3 on web page 450 of his article shows the outcomes for 9 batsmen, together with some in every of those three classes.
Kimber says that in instances of a large proportion of Not Out innings his methodology “isn’t any extra wise than the conventionally estimated common”. The very best proportion of Not Out Innings for these 9 batsmen is 26%. He doesn’t really specify an higher threshold, a big level returned to shortly.
Comparability of Kimber’s Reported Outcomes with Conventional Averages
The ensuing batting common utilizing Kimber’s methodology will often, although not at all times, be smaller than a batsman’s Conventional Common. The result relies on the batsman’s profile of scores. Nonetheless, a substantial discount will happen for a big proportion of First Class batsmen. A typical case is a participant with pretty excessive proportion of Not Out Innings who doesn’t make many very excessive scores. Supplied {that a} batsman’s highest Accomplished Innings Rating is bigger than his highest Not Out Rating, it follows that Kimber’s ensuing common can’t exceed the previous, which is a sound reasonableness test.
Kimber experiences outcomes for the next batsmen: Mohinder Amarnath in Checks (often coming in at quantity 3), Don Bradman in Checks and in all First Class matches, David Gower in Checks (200 of his 204 innings as much as time of study), Victor Trumper in First Class matches, and 4 Surrey gamers for the 1983 English County Championship season – Monte Lynch, within the center order; Alec Stewart, often at quantity 3; Jack Richards (wicket-keeper) within the decrease center order; and Sylvester Clarke, a tail-ender.
| Conventional Ave | Kimber’s Ave | |
| Amarnath (113 inns, 8.8% not out) | 42.50 | 41.40 |
| Bradman (80 Check inns, 12.5% no) | 99.94 | 98.98 |
| Bradman (338 FC inns, 12.7% no) | 94.76 | 95.14 |
| Gower (200 inns to mid-1992, 8.0% no) | 44.31 | 44.30 |
| Trumper (395 inns, 5.3% no) | 46.58 | 47.36 |
| Lynch (39 inns, 25.6% no) | 53.72 | 49.11 |
| Stewart (16 inns, 18.8% no) | 31.31 | 30.71 |
| Richards (34 inns, 23.5% no) | 27.61 | 27.46 |
| Clarke (24 inns, 16.7% no) | 14.25 | 14.15 |
Kimber’s adjusted common is decrease most often, although it’s a little increased for Bradman and Trumper for his or her First Class matches. Aside from Lynch (down 8.6%), although, the modifications are fairly small, with solely the distinction for Amarnath exceeding 2.0%.
Afterword
Rounding off their article, Kimber-Hansford put ahead a proposal to increase the statistics of the variety of 50s and variety of 100s made by a batsman as often offered in abstract information on careers in numerous codecs of the sport. Whereas indicating the variety of instances a batsman reaches or passes these two milestones, this apply stops wanting informing the reader in regards to the nature of the “previous” bit.
Their proposal is to offer a abstract description of a batsman’s distribution of scores, they usually counsel highlighting his 50th, 75th and 90th centile scores (the precise alternative of centiles being arbitrary). They illustrate what this suggests for a collection of batsmen at First Class stage, noting that the tenth and 25th centiles are low for the nice majority of batsmen. (The 25th centile being one-quarter up the checklist of a batsman’s scores, working from the smallest to largest.)
Returning to Danaher’s article, though substantial elements of his textual content are inconceivable for a lay particular person to comply with, he illustrates the applying of the Product Restrict Estimator (PLE) to the innings of six gamers in England’s county championship, 4 of whom had an unusually excessive proportion of Not Out Innings. Deploying my simplified model of the PLE mechanism, I’ve replicated his discovering for Martin Crowe (29 innings for Somerset in 1987 at an estimated common of 63.75). Danaher sees the principle deserves of utilizing the PLE for deriving batting averages as twofold:
- producing a extra wise rating of gamers’ comparative skills, and
- rectifying anomalies with tail-enders’ averages, whereas nonetheless rewarding Not Out innings.
Regardless of the rigour and inherent enchantment of the PLE method, there isn’t any demonstrably appropriate resolution to finishing partial information, and it’s uncommon for the outcomes of doing so to be examined afterwards. And in batting, there isn’t any risk – even in precept – of verification!
Subsequent Notables
Two very totally different strategies at the moment are mentioned, that are these developed and utilized by Lemmer and by Maini/Narayanan.
Professor Hermanus (“Hoffie”) Lemmer of the College of Johannesburg is unquestionably the doyen of these making use of statistical strategies to analyse cricket information and participant efficiency, having had no less than 23 such papers revealed in the course of the interval 2001-17.
His Focus and Kimber’s Boundary
Lemmer tackles the issue that Alan Kimber left untouched. Recall that when a batsman’s proportion of Not Out Innings (Prop NOI) reaches a sure stage, Kimber’s methodology of arriving on the “true” common is, in his personal phrases, “no extra wise than the conventionally estimated common”. To anticipate what comes later in additional element, Lemmer finds that when Prop NOI reaches 40% for batsmen within the ODI format of the sport, Kimber’s methodology turns into insufficiently dependable, and is more and more unreliable as this proportion enters increased ranges. The identical downside applies to Check matches when Prop NOI turns into “a lot higher than round 20%”; and I assume right here that this threshold will be put at roughly 23% (ie 15% increased than 20%). Kimber’s unreliability at these levels is as a result of too little reliance is being positioned on Accomplished Innings for projecting Not Out Scores to a notional conclusion.
It’s this challenge of discovering an acceptable methodology when Prop NOI is increased than a essential stage to which Lemmer’s extensively cited 2008a article is directed. His proposals are subsequently to be considered filling the void that Kimber left in his wake. They’re primarily a complement to Kimber’s methodology, so making it extra full.

Hoffie Lemmer – Revolutionary Methodology to Fill a Void
Lemmer’s proposals take the type of two “Estimators” of the batting common. They’re specified as formulae, although are ones that may readily be understood by those that don’t have a grounding in statistical issues. Considered one of these Estimators is for common software to restricted overs matches, the opposite for common software to Check matches and different limitless overs matches when a batsman’s Prop NOI reaches/exceeds the thresholds simply famous. For every of those codecs of the sport, the components itself doesn’t alter in any approach for various batsman, despite the fact that the info wanted to use it are distinctive to every batsman. The information inputs are primary statistics, such because the sum of a batsman’s dismissal scores, the sum of his not out scores, the typical of his not out scores and the proportion of innings through which he has remained not out. These will be simply derived from customary information on web websites reminiscent of cricinfo and howstat.
It’s important that Kimber’s reported findings for all of his 9 batsmen relate to these with Prop NOI effectively underneath Lemmer’s two markers. Particularly: Kimber’s 5 batsmen in limitless overs matches have Prop NOI no higher than 13%, and his 4 batsmen enjoying for Surrey County in three day, two innings a aspect, matches have Prop NOI that’s no higher than 26%. The latter championship matches are extra akin to restricted overs matches than to Checks in view of their time-scale, particularly as unhealthy climate has usually decreased enjoying time by no less than half a day. (In all, 13 of Surrey’s 24 championship matches that season had been drawn. Three of those attracts ended with no multiple and a half of the potential 4 innings being performed, and one other three attracts ended with no extra two and a half innings being performed.)
At this juncture, it’s essential to probe a possible disconnect between the respective statements of Kimber and Lemmer. When mentioning the boundary for the proportion of Not Out Innings making use of to his personal methodology, Kimber makes use of the time period “large”, whereas Lemmer makes use of the time period “massive” (proportion) as the main target of his Estimators. To inform whether or not Lemmer’s markers of 40% and 23% are in battle with Kimber’s said restrict, “large” needs to be interpreted as Kimber didn’t remark additional. There are two methods he could have checked out it.
The primary pertains to an summary scale, starting from, say, zero as much as a most of 100%, with all ranges being equally more likely to be reached. A proportion of 90% or 95% and upwards may then be taken as representing his (and most of the people’s) thought of what represents large. Nevertheless it appears extra possible that Kimber considered large throughout the context of batting statistics and his appreciation of what’s a uncommon or uncommon proportion of Not Out Innings – the next observations being pertinent:
ODIs and their ilk
- Since ODI matches started in 1971 via to mid-December 2021, there are 113 England gamers with a minimal of 14 innings: of those, eighteen attain or exceed the brink of 40% NOI – that’s 16%. All besides three of the eighteen are tail-enders. (Solely three of the gamers, all being tail-enders, have Prop NOI of fifty% or extra.)
- For all nations since 1971 via to mid-December 2021, there have been 99 gamers with a minimal of 20 innings and an official common of no less than 38.0 and so they’re specialist batsmen or real all-rounders. Of those, simply 1 participant has Prop NOI exceeding 40% (Imad Wasim of Pakistan, at 42.5% for 40 innings – the subsequent highest is 36.5%, making use of to 2 gamers).
- Taking the three day matches of the 1983 English County Championship, which Kimber drew on: of the 205 gamers with a minimal of 14 innings, solely 6 of them – simply 3% – have Prop NOI reaching/exceeding 40%. These are all tail-enders.
This proof means that Lemmer’s 40% marker isn’t met within the restricted overs format, besides within the case of tail-enders and even for them that is an uncommon incidence.
Check matches and their ilk
- Within the case of England gamers since Checks had been initiated via to mid-December 2021: of the 229 gamers with a minimal of 20 innings, there are 32 – ie 14% – with Prop NOI reaching or exceeding 23%. All of them are tail-enders. (An additional 6 gamers have Prop NOI of 20-22%, all besides two being tail-enders.)
- Taking the 4 day matches of the 2018 English County Championship, Division 1: of the 64 gamers with a minimal of 14 innings, solely 5 – ie 8% – have Prop NOI reaching/exceeding 23%.All these are tail-enders. An additional three gamers have Prop NOI of 20-22%, and once more they’re tail-enders.
This means that assembly Lemmer’s 23% marker is extraordinarily uncommon for specialist batsmen and all-rounders, and even for tail-enders it’s uncommon.
In conclusion, Kimber and Lemmer are not basically in battle over this boundary challenge. Defusing it has been well worth the effort and size of exposition concerned as an adjudication would in any other case have been wanted!
Roles that Lemmer’s Estimators Can Play
For these wanting to use Lemmer’s Estimators of a “true” common to batsmen’s entire careers, these clearly have a really restricted position to play apart from for tail-enders. However for a brief collection of matches, his contribution ceases to be of a distinct segment nature and has a large software. Examples are:
- The ODI and Twenty20 World Cup competitions – respectively giving every batsman as much as 11 innings and seven innings.
- A single collection of ODI or Check matches, the latter sometimes having potential for 6-10 innings.
- The Royal London One-Day Cup competitors: for season 2021, producing 11 innings within the case of 1 batsman, the subsequent highest being 9 innings.
- A season within the Indian Premier League which supplies for as much as 14 innings, plus the play-off matches.
In a single specific quick collection, the inaugural Twenty20 World Cup competitors, Lemmer utilized his really useful Estimator to plenty of innings performed as little as three (2008b article).
In deriving his really useful Estimators, Lemmer employs customary statistical methods – aside from one ingenious transfer that I shall draw consideration to in the end. What’s of relevance right here is the underlying logic which I attempt to lay naked within the following sections. That is wanted if a lay particular person is to have faith within the outcomes to be obtained from their software.
Why Kimber’s Methodology Produces Unstable Outcomes, at Some Stage
The primary and essential factor to uncover is why it must be that Kimber’s methodology of figuring out a “true” batting common turns into unreliable when Prop NOI reaches a sure stage, having labored satisfactorily earlier than then. (Recall that his methodology centres on projecting Not Out Scores to a notional completion by taking the mathematically anticipated worth of all of the scores that exceed or equal it.) This may be defined, at an intuitive stage, by noting that the variety of related scores on which to base the projections of Not Out Scores (NOSs) turns into fewer and fewer as a batsman’s Prop NOI will increase.
Sooner or later, additional small reductions within the variety of related scores have a big and erratic impact on the ensuing projection, resulting in the estimated “true” common turning into unstable. This displays the tendency for the accessible scores on which to mission NOSs then turning into sparse and patchy.
The comparatively massive impact of a small change in variety of Dismissal or Not Out innings is seen within the case of Steven Finn’s Check match profession of 47 innings and Prop NOI of 47%. Deleting two Dismissal Innings of scores of 17 and 19 runs (in a beginning whole of 25 Dismissal Innings and 213 runs) results in a rise of 8% in his whole projected Not out Scores. And deleting one Not Out Innings, of a rating of 16 (in a beginning whole of twenty-two Not Out Innings) produces a 5% discount to his estimated “true” common.
Principal Options of Lemmer’s Methodology
As an alternative of utilizing the uncooked information on scores to drive the evaluation, as Kimber did, Lemmer works with a statistical curve to symbolize the distribution of a batsman’s scores (from excessive to low). In abstract: working with rigorously chosen samples of ODI batsmen and Check batsmen, he in the end combines the scoring information for essentially the most appropriate 9 batsmen for every of those codecs, having first projected all the Not Out Scores to a notional conclusion. The set of aggregated information on ODI scores – and the mixture information on Check match scores – can then be considered regarding a single “composite” batsman in every case.
Lemmer matches a curve to the mixture information on ODI scores, discovering the form of curve that provides the closet match to the info set. Given the precise form of the curve, its Imply worth (ie the arithmetic common) will be discovered utilizing a normal rule. This worth represents the “true” batting common for his assortment of 9 ODI batsmen. The ultimate step is to find out a exact components that provides the identical reply as that Imply worth, or a really shut approximation to it. That is his “Estimator” for common use when an ODI batsman’s Prop NOI reaches/exceeds the 40% threshold. The identical process is {followed} with the mixture information on scores for the 9 Check match batsmen.
The Estimator for every format of the sport can then be utilized to a given batsman irrespective of any fitted curve and rely, as talked about earlier, on a number of available efficiency statistics.
| For these wishing to know one thing of the supporting element, the principle steps are elaborated on under. In any other case, the reader can skip forward to the sub-heading “Particular Type of the Advisable Estimators”, after which the impact of making use of the Estimators is examined and in contrast with the outcomes of utilizing Kimber’s methodology. |
The Samples of Batsmen – ODIs and Check Matches
Lemmer works with random samples of specialist and all-rounder batsmen, drawn from your entire pool of then present gamers (as at mid-August 2005), spanning all taking part nations. All are effectively established gamers and practically all have reasonable to sturdy official averages (the bottom being within the twenties).
For ODI matches, he works initially with 22 batsmen who, with simply two exceptions, have performed no less than 100 innings. He wants a excessive variety of scores for every batsman to be able to make his statistical evaluation sufficiently dependable for sensible software. Specifically, this allows a great match to be achieved when superimposing a normal kind of statistical curve on the scores made by a given participant. The 5 gamers with the best Prop NOI are within the 30-37% vary; the subsequent 4 gamers spanning 20-29%, with a lot of the different 13 gamers spanning 8-18%.
For Check matches, Lemmer works initially with the scores for 20 skilled batsmen, every with no less than 100 innings (starting from 103 to 179). General, their Prop NOI are a lot decrease than for ODIs – the best six being within the vary of 13-19%., lowering right down to solely 5%.
In projecting every of a batsman’s NOS to notional conclusion – known as an augmented or full rating – Lemmer has regard to scores made all through the batsman’s profession. He converts every NOI into an entire rating by taking the mathematically anticipated worth of these scores.[viii] Every related rating is assigned an equal chance of occurring. (Accordingly, if there are three innings performed every of 25 runs, the rating of 25 is given 3 times the chance of a rating of 35 runs made in just one innings.) This quantity to precisely the identical factor as taking the Imply worth of all of the separate particular person scores that equal or exceed the NOI in query.
In doing this, account is taken of different Not Out Scores at their projected values. It’s famous that Lemmer’s process for projection produces an an identical outcome to Kimber’s methodology of doing so utilizing the PLE mechanism (as described earlier).
Every Not Out rating is changed by the “augmented” rating and included alongside all really accomplished scores to offer a single set of scores for every batsman, as a foundation for Lemmer’s curve becoming train.
Becoming Statistical Curves to Batsmen’s Scores
In abstract, Lemmer superimposes a statistical curve on the array of a batsman’s ODI scores (organized from excessive to low) and, individually, does this additionally for the array of a batsman’s Check match scores. Quite a few potential shapes of curve are tried out and from these the one that almost all carefully matches the scores information is recognized.
Becoming such a curve to batting scores acts as a pattern line via the distribution. In impact, it supplies a smoothed model of the uncooked information on particular person scores. So the precise distribution of scores made will depart considerably from the fitted curve – deviating upwards from the curve alongside some elements, downwards alongside another elements. Additionally, whereas the fitted curve will include a steady collection of information factors, with the uncooked information collection there will probably be some discontinuities alongside the development of sores made (from excessive to low). These two kinds of disparity received’t be of fabric consequence as long as the match to the info is shut.
Attaining a really shut matching to the uncooked information on a batsman’s scores, in order that the curve is an excellent illustration, is important for Lemmer’s purpose of arriving at dependable Estimators of the batting common – as he himself stresses.
To elaborate: statistical curves of various sorts are fitted to the scores of every batsman and are then assessed for matching. These are mainly curves of the so-called Gamma and Weibull households. Examples of the Gamma household are proven under, being extensively utilized in numerous fields of science to mannequin information on variables which have skewed distributions – as sometimes applies to batting scores.
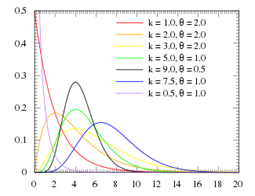
A putting characteristic of each the Gamma and Weibull households is the very totally different shapes these distributions can take. Their versatility means there’s sturdy potential to offer a great approximation to typical patterns of batting scores. The 2 left-to-right downward sloping examples of the graph are of potential relevance.
The very best form of curve for every batsman is established by making use of a normal statistical method often called “most chance estimation”. The precise form it takes relies on the worth specified for its two parameters.
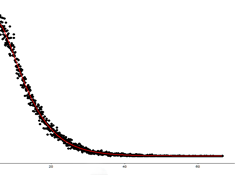
Instance of a fitted Gamma kind curve and the uncooked information on scores
The comparative efficiency of the perfect Gamma curve and the perfect Weibull curve for every batsman was assessed by establishing the utmost distance between the scores made and the fitted curve – smallest distance being greatest. Lemmer finds {that a} Gamma kind of curve is, in general phrases, greatest for each his ODI and Check match batsmen. The precise form it takes varies from one batsman to a different, reflecting the variation of their scoring profiles.
The ensuing “true” averages for the 2 samples of batsmen had been derived instantly from a given batsman’s fitted curve by dividing the worth for one in all its two parameters by the worth for the opposite. Lemmer finds that the estimated batting averages have a really shut correspondence with these obtained by an alternate methodology that makes no use of statistical curves, offering that the proportion of Not Out Innings for the batsmen involved is effectively under his essential threshold ranges (40% for ODIs and round 23% for Check matches). The choice is solely to take a batsman’s set of projected Not Out Scores, add these to his Dismissal Scores after which divide via by the full variety of innings performed. This correspondence justified a excessive confidence being positioned within the curve-fitting method going ahead when excessive proportions of Not Out Innings are thought of.
A Dilemma and an Ingenious Answer
Nonetheless, Lemmer finds himself on the horns of a dilemma as a result of the requirement for having numerous innings performed by the assorted batsmen comprising his samples was incompatible with desirous to discover a appropriate Estimator for Prop NOI of 40% (and upwards) for ODIs and of round 23% (and upwards) for Check matches. As his two samples are comprised of entire careers of specialist batsmen and real all-rounders, it was inevitable that their Prop NOI would fall wanting these markers. For these batsmen with the perfect curve matches (9 of the 22 for ODIs, and 9 of the 20 for Check matches), the best Prop NOI is just 24% within the case of ODIs and solely 17% for Check matches. And it’s these batsmen who’re retained for the remainder of his research, exactly due to their excellent curve matches. (Every of them had performed no less than 160 ODI innings or no less than 130 Check match innings.)
Giving up both the composition of his chosen 9 ODI and 9 Check match batsmen or his related thresholds was not solely extremely undesirable, it will have been self-defeating! And Lemmer couldn’t use information on a brief collection of matches to provide batsmen with the specified Prop NOI as a result of they wouldn’t have performed sufficient innings to realize excellent curve-fitting and dependable outcomes![ix]
Lemmer resolves this dilemma in an ingenious approach. For every of the chosen 9 ODI batsmen and 9 Check match batsmen, he transforms a adequate variety of their Dismissal Scores into equal supposed Not Out Scores, in order to realize the specified 40% and 23% Prop NOI. He does this “down-scaling” for a given batsman by making use of a few of his personal ratios of precise Not Out Scores to their projected accomplished scores (one specific ratio, or relationship, making use of to every NOS). Each the actual Dismissal Scores to be reworked, and the ratios to be utilized to them, are chosen in a random method.
For instance: within the case of Abdul Razzaq (one of many 9 chosen ODI batsmen), 67 Not Outs scores are required however he has solely 41 precise Not Out scores, and so 26 of his 127 Dismissal Scores should be down-scaled. Every reworked rating is subsequently scaled again up utilizing the inverse of the down-scaling ratio, thereby being returned to its unique worth. All of the chosen batsman will now have an acceptable, revised, set of scores that Lemmer can work with. Every of them will get a revised curve fitted to their scores as their unique Not Out Scores have been projected in another way, there being fewer related Accomplished Innings Scores on which to base the projections.
Though this down-scaling train does appear to have logic on its aspect, a lay particular person will be forgiven for considering that now we have now stepped into Alice in Wonderland territory – Lewis Carroll’s story, revealed in 1865, which nonetheless shines as a part of the literary nonsense style.[x]
Discovering Dependable Estimators of the Batting Common – for ODI & Check match scores
One other key transfer was now made by Lemmer. This was to pool the set of information for the 9 chosen ODI batsmen and likewise for the 9 chosen Check match batsmen. In every case, a greatest match curve was utilized to the scores of the 9 batsmen collectively – ie to their mixture information – so {that a} usually relevant Estimator could possibly be recognized. With out such pooling, totally different Estimators would have emerged for the person batsmen and no concrete suggestions for arriving at “true” averages may have been formulated. (Pooling additionally, by the way, countered the tendency for the scores made on the excessive finish of the batsmen’s distributions to be thinly unfold or patchy.)
For every of the 2 codecs, quite a few different potential Estimators had been formulated and evaluated in accordance how effectively they mirrored the form of the batsmen’s collective curve, this being completed utilizing a standard statistical check (the root imply sq. error criterion).
Lemmer’s three greatest Estimators for every format, which all carried out “reliably”, had been then in contrast with Kimber’s Estimator and the Conventional Common components. Lemmer’s three are proven to decisively out-perform the opposite two on the 40% and 23% NOI markers, the extra so with nonetheless increased proportions of Not Out Innings. His personal perfect Estimator for every format carried out “very reliably”.
Particular Type of Lemmer’s Advisable Estimators
For ODIs and different restricted overs matches
The Estimator model known as “e6” was discovered to be essentially the most appropriate for these matches, in addition to being simple for non-specialists to calculate. It takes the shape specified under.
Sum of Dismissal Scores plus (f6 instances the Sum of Not Out Scores),
divided by Variety of Innings performed.
through which f6 = 2.2 – (0.01 instances the typical of Not Out scores)
The image f within the components is the issue by which a Not Out Rating is scaled as much as get hold of a notionally accomplished rating. For example, if the typical of a batsman’s Not Out Scores is 35, f would have a price of 1.85 (2.2 minus (0.01 instances 35)) and his Not Out Scores, totalled up, could be multiplied by that quantity after which added to the full of his Dismissal Scores. The ensuing reply is then divided by the full variety of innings performed to offer his “true” common.
For Checks and different limitless overs matches
Essentially the most appropriate Estimator for these matches was discovered to be “e8”, which is given as:
Sum of Dismissal Scores plus (f8 instances the Sum of Not Out Scores),
divided by Variety of Innings Performed.
through which f8 = 2.2 minus (0.01 instances the typical of Not Out Scores) plus
(0.15 instances the proportion of Not Out Scores).
The proportion of Not Out Scores is expressed as 0.15 (for 15%), 0.35 (for 35%), and so forth.
Observe that the relative emphasis positioned on the person parts of the 2 formulae varies, and so their respective diploma of affect on the ensuing common differs.
Most notable within the class of limitless overs matches, in addition to Checks, are Australia’s inter-state competitors, West Indies regional competitors (previously the Shell Defend), South Africa’s inter-provincial competitors and, from 1993 onwards, the English County Championship – all these matches being performed over 4 days and therefore of comparable period to Check matches (three to 5 days).
Impacts on Batting Averages In contrast with Kimber’s Methodology
Restricted Overs matches
Of their evaluation paper of 2011, Paul van Staden and colleagues (College of Pretoria) look at what Lemmer’s “e6” components and Kimber’s methodology estimate for a bunch of 9 gamers who participated within the restricted overs format of the 2010 Indian Premier League. These batsmen every had between 7 and 16 innings, together with no less than one Not Out innings.
Three of those gamers had their proportion of Not Out Innings (Prop NOI) above 40% (Lemmer’s marker): Mithin Manhas at 50%, and Kevin Pietersen and Adam Voges at 43%. Each Lemmer’s and Kimber’s methodology gave outcomes decrease than the Conventional Common.[xi]
Lemmer’s ensuing averages are increased than these of Kimber for all 9 gamers, the next factors being of curiosity:
- The distinction between the 2 units of averages tends to get bigger (in share and absolute phrases) as Prop NOI will increase.
- For the 4 instances of Prop NOI between 23% and 31%, Lemmer’s ensuing averages work out considerably increased than for Kimber (one-sixth increased).
- For 2 of the three batsmen with Prop NOI of no less than 40%, the variations are massive: Lemmer’s common being 32% and 39% increased than for Kimber. Within the different case, that of Kevin Pietersen, the distinction is small (Lemmer’s being 6% increased than Kimber’s). It is a reflection of the truth that Pietersen’s prime rating was a Not Out and his two different Not Outs had been his third and fourth highest scores in a usually low scoring collection of seven innings.
Three day, two innings a aspect matches (akin to ODIs); and Check matches
For the seven batsmen that Kimber reported his findings, their respective Prop NOI are effectively under Lemmer’s thresholds, and so comparisons of batting averages utilizing their two strategies are of restricted curiosity. Suffice to say that the 2 units of averages derived for six of those seven batsmen are fairly shut, all being inside one run per innings and three of them being inside 0.4 run per innings.
This closeness displays the truth that their respective ratios of Not Out Scores to projected completion for these batsmen are fairly comparable. Lemmer’s multiplier ranges from 1.77 to 2.08 for the 4 batsmen in ODI kind matches, and from 1.39 to 1.60 for the three Check match batsmen; whereas Kimber’s multipliers for these two teams vary, respectively, from 1.75 to 1.92 and from 1.49 to 1.82.
The overall nearness to a multiplier of two.0 is noteworthy and reassuring. In his 2008a article, Lemmer contends that underneath the next assumption – that the exterior elements which may curtail a batsman’s innings with out him being dismissed are random and unbiased of his doubtlessly accomplished rating – then, on common, he could possibly be anticipated to have doubled his undefeated rating.
Though Lemmer doesn’t provide the related reasoning, an anticipated common multiplier of round 2.0 could also be defined on this approach. Given a really massive variety of Not Out Innings and in addition of Accomplished Innings, the distribution of a batsman’s (stranded) Not Out Scores would possible vary from an approximation to the common of his Accomplished Innings Scores (ie being totally fulfilled) via to his lowest Accomplished Innings Rating which is often zero (being wholly unfulfilled). With very quite a few innings performed, a reasonably even distribution of Not Out scores could possibly be anticipated between these two values, with an general Imply worth at round half the typical of the batsman’s Accomplished Innings Scores. Therefore, Not Outs would, in mixture, be doubled to achieve whole completion scores.[xii]
Turning now to Sanchit Maini and Sumit Narayanan, two skilled actuaries who diverted from their regular work to provide a novel methodology to appropriate for the defects they perceived with the normal batting common. Their proposed methodology is defined in a two web page piece revealed in 2007.
Having aired their mistrust of the Conventional Common as a dependable measure of central tendency – ie as being consultant of a batsmen’s general distribution of scores – these authors suggest a way of arriving at batting averages that pulls on an analogy with the idea of publicity to danger, as utilized on the planet of insurance coverage. This methodology does have its sights and is usually cited within the literature, even getting publicity in The Economist journal.
In essence, Not Out Innings (NOIs) are transformed into plenty of accomplished innings equivalents by figuring out the variety of deliveries confronted and evaluating that quantity with the variety of deliveries confronted per accomplished innings when taken over the batsman’s entire profession. For comfort, I consult with the latter because the batsman’s “profession grand common”. (The authors don’t really use the time period accomplished innings. But if all innings is utilized as an alternative, their resolution would usually make little sense. So I assume the previous does apply.)
I’m glad with the entire profession foundation (both completed or nonetheless in progress), fairly than variety of innings performed as much as the incidence of a NOI in query, for causes given in Half II the place this challenge crops up acutely.
A batsman’s profession grand common is denoted by 1.0. Every NOI has some extent of related publicity to danger, and is given a proportional fraction of 1.0 if the variety of deliveries confronted is lower than his profession grand common. These professional rata fractions are added collectively to offer the batsman’s variety of equal accomplished innings.
If the variety of deliveries confronted in a NOI is equal to, or higher, than his profession grand common, it’s accorded a full 1.0 – equating to 1 accomplished innings which then contributes to the denominator, ie his variety of precise and equal accomplished innings. Complete runs scored are then divided by that variety of innings to reach on the common that represents his demonstrated functionality.
Accordingly, the diploma of danger publicity for a NOI is capped on the batsman’s profession grand common for the variety of deliveries confronted in accomplished innings. To assign a price of higher than 1.0 if he survives higher danger publicity than his profession grand common could be perverse, as it will penalise him for an above regular stint of survival. I’m uneasy about the truth that a batsman who survives past his profession grand common and stays Not Out is, nonetheless, handled as having performed a “full” innings for calculating his consultant common.[xiii]
Maybe a approach round this downside could be to establish the typical variety of deliveries acquired in these accomplished innings that equal or exceed the batsman’s profession grand common, after which state the NOI in query as a fraction of that quantity. If there are a number of innings of this nature, the fractions could be added.
The Maini/Narayanan methodology has the advantage of rewarding comparatively quick scoring in enjoying a Not Out Innings, although it penalises a batsman for sluggish scoring which can have been justified, as in attempting to stave off defeat or going through excessive class bowling.
Sadly, regardless of its sights, the proposed method can’t be carried out for a lot of former batsmen because the variety of deliveries confronted has usually not been recorded, or no less than not preserved, for Check matches (not to mention different First Class matches) – such because the West Indies versus India collection of 1952/53, England versus West Indies in 1957, India versus England in 1961/62 and Sri Lanka versus Pakistan in 1986.
NOTES
[i] With the normal calculation of a batting common, the full of the Not Out Scores (NOSs) are unfold throughout all the Accomplished Innings Scores – eg a NOS of 8 runs could also be unfold over 4 accomplished innings totalling 30 runs, so including a premium of an extra 2 runs to a Accomplished Innings Common of seven.5 to offer 9.5. That is equal to projecting that NOS to a Dismissal Rating of 17.5 runs, giving a complete of 47.5 runs for what at the moment are 5 “accomplished” innings (so sustaining the said general common of 9.5). When a Not out Rating is projected to a completion, that in impact will increase the variety of accomplished innings by one, and so the premium needs to be utilized to the projected NOS as effectively.
[ii] This hardly ever occurs in apply; when it does it’s often for a batsman who has performed as much as round half a dozen innings.
[iii] The shape utilized is termed exponential smoothing as exponentially lowering weights are used as one strikes ahead throughout the distribution of plotted accomplished scores from low to excessive (or excessive to low). This method is usually used for evaluation of time-series information.
[iv] The 12 units of scores had been for Allan Border, Ian Botham, John Emburey, Gordon Greenidge, Kim Hughes, Viv Richards and Jeff Thomson in Checks; and for David Gower, Desmond Haynes, Imran Khan, Javed Miandad and Kris Srikkanth in ODIs.
[v] This text was a prolonged time within the making, when Kaplan was at Bell Phone Laboratories in New Jersey and Meier was at John Hopkins College in Baltimore. Independently, they did associated analysis starting in 1952/53 and submitted separate manuscripts to the Journal whose editor persuaded them to provide a joint (merged) model. Correspondence spanning plenty of years was required to reconcile considerably totally different approaches to addressing the issue.
[vi] The analogy is presumably with censorship within the arts and literature, very like some traces in a stage play being suppressed by a authorities official and so are lacking from the reside efficiency.
[vii] Kimber’s modelling is equal to changing a prime rating Not Out Innings to an estimated accomplished rating by including on the general common of all of a batsman’s scores. My suggestion is a refinement that nullifies the excessive danger sometimes confronted early on in an innings.
[viii] This was deciphered for me by Hoffie Lemmer, making reference to the formidable wanting equations given in his 2001 article (Section2, web page 46), from which was derived the abstract equation on web page 69 of his 2008a article.
[ix] If Lemmer had chosen tail-enders, most of them could have had NOIs of 40% plus, however he would then be unable to check the reliability of utilizing curve becoming in opposition to the choice methodology simply famous.
[x] The down-scaling undertaken appears to be no much less outlandish than, as an illustration, a college that has too few undergraduate college students to qualify for an uplift in its authorities grant, using a time machine to transform a lot of its post-graduates into their former pre-graduate selves. I’ll go away the reader to think about parallel examples throughout the context of Carroll’s fantasy story.
[xi] Of their evaluation article of 2016, P.Ok. Gaur and D. Bhattacharjee (Assam College, India) look at the estimated batting averages that Lemmer’s “e6” model (and another proposals) produces for a bunch of 20 gamers who participated within the 2015 ODI World Cup (performed in Australia and New Zealand). Nonetheless, for none of those gamers does the proportion of Not Out innings attain Lemmer’s marker of 40% – the closest being M.S. Dhoni at 30%, and A.B. de Villiers and Ok. Sangakkara at 29%.
[xii] There’s a supportive analogy right here with software of the rule-of-a-half utilized by economists in valuing the time saved by these making further car journeys following a freeway enchancment scheme. Within the absence of empirical proof, the additional journeys so generated are assumed, on common, to provide a profit approximating to half of the time financial savings accruing to the base-load of journeys (ie these made previous to the advance in journey circumstances.) At most, an induced traveller will be anticipated to learn on the full extent skilled by the base-load travellers, and at minimal by a minimal quantity. The reasoning is that some further journeys could be induced even by a really small discount in journey time while, on the different excessive, some journeys would solely simply be induced by the total discount made to journey time; and it’s assumed there’s a steady and even distribution of induced journeys between these two limits.
[xiii] Though a batsman is partly rewarded for his longer survival via any further runs he makes, that is inappropriate (and, in such a case, he would possibly really make fewer runs than when enjoying innings through which he has confronted lower than his profession grand common).
REFERENCES
P. Barton: Do Tail-Enders’ Batting Averages Profit From Not-Outs? The Cricket Statistician Journal, Autumn 2015 (pp 18-20).
P.J. Danaher: Estimating a Cricketer’s Batting Common Utilizing the Product Restrict Estimator.
The New Zealand Statistician. June 1989 (pp 2-5).
Ok. Date: The Calculus of the Batting Common. Cricinfo web site, 29 Might 2014 (6 pages).
I. Etikan, S. Abubakar and R. Alkassim: The Kaplan-Meier Estimate in Survival Evaluation.
Biometrics & Biostatistics Worldwide Journal, February 2017 (pp 55-59).
H. Ganjoo: Learn how to Account for Not-Outs Extra Precisely When Assessing Batsmen.
Cricinfo web site, 27 Might 2018.
P.Ok. Gaur and D. Bhattacharjee: On Discovering the Most Suitable Batting Common.
Journal of Utilized Quantitative Strategies, September 2016 (pp 50-60).
B. Kachoyan and M. West: Deriving an Actual Batting Survival Perform in Cricket.
14th Australasian Convention on Arithmetic in Sport, July 2018.
E.L. Kaplan and P. Meier: Nonparametric Estimation from Incomplete Observations.
Journal of the American Statistical Affiliation, 1958 (pp 457-81).
A.C. Kimber and A.R. Hansford: A Statistical Evaluation of Batting in Cricket.
Journal of the Royal Statistical Society, 1993 (pp 443-55).
H.H. Lemmer: Estimation of the Batting Potential of South African Worldwide One-Day Cricket Gamers. South African Journal of Science and Expertise, Challenge Quantity 2, 2001 (pp 45-48).
H.H. Lemmer (2008a): Common Measures of Batting Efficiency in a Quick Sequence of Cricket Matches. South African Statistical Journal, Challenge No. 1, 2008 (pp 65-87).
H.H. Lemmer (2008b): An Evaluation of Gamers’ Performances within the First Cricket Twenty20 World Cup Sequence. South African Journal for Analysis in Sport, Bodily Schooling & Recreation. Challenge No. 2, 2008 (pp 71-77).
S. Maini and S. Narayanan: The Flaw in Batting Averages. The Actuary (UK journal),
Might 2007 (pp 30-31).
H. Saikia and D. Bhattacharjee: Survival Potential of Indian and Abroad Batsmen on the Cricket Pitch in Indian Premier League. MOJ Sports activities Drugs, Challenge 4, 2018 (pp 113-16).
P.J. van Staden, A.T. Meiring, J.A. Steyn and I.N. Fabris-Rotelli: Significant Batting Averages in Cricket. South African Journal for Analysis in Sport, Bodily Schooling & Recreation. January 2011 (pp 75-82).
G.H. Wooden: Cricket Scores and Geometric Development. Journal of the Royal Statistical Society,
1945 (pp 12-22).
[ad_2]
Source link


